Aleksey V Ilyin
Moscow Institute of Physics and Technology, Russia
Title: On some unknown features of the Bose-Einstein statistics
Biography
Biography: Aleksey V Ilyin
Abstract
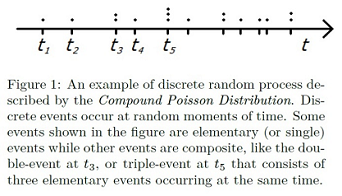
It is proven within the Bose-Einstein statistics that the blackbody radiation contains photon clusters along with single photons. The existence of photon clusters in blackbody radiation is shown to be an inevitable consequence of the fact that the Bose-Einstein statistics is a special case of Compound Poisson Distribution. This mathematical fact means that the BE statistics describes random events of two different types: elementary events and composite events, each composite event consisting of a random number of elementary events. In the case of photon statistics, an elementary event may only be the registration of a single photon by an ideal detector. Then a composite event will be a simultaneous registration of several photons, i.e. of a photon cluster. In this work, statistics of photon clusters and probability that a photon cluster contains N photons are found versus radiation frequency and temperature. Spectra of photon-cluster radiation in a blackbody cavity are calculated for arbitrary cluster ranks. Derivation of the Planck's radiation law is discussed in view of the existence of photon clusters in blackbody radiation. Finally, we analyze some properties of photon clusters. It is proven that the behavior of photon cluster field is incompatible with the Maxwell’s equations for electromagnetic radiation. Therefore, a photon cluster field cannot obey the Maxwell’s equations. A decades-long search for suitable equations carried out by many researchers is reviewed. Strong arguments are presented in favor of the conclusion that a 2-photon cluster field is a rank-2 tensor field that must obey the Einstein field equations of general relativity. For that reason, a 2 photon cluster field must propagate as if it were an optical-frequency gravitational wave.